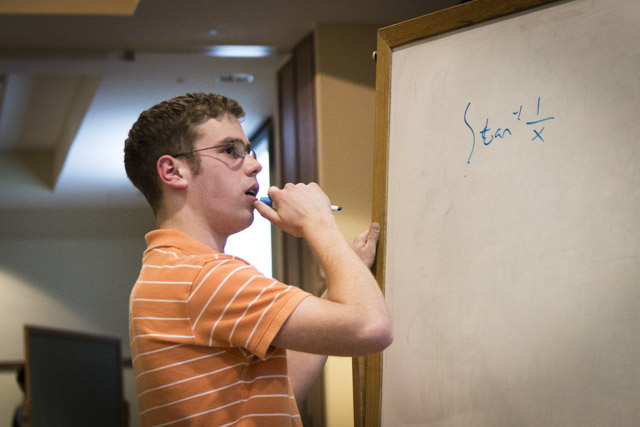Integration Bee

The 2025 High School Integration Bee is scheduled for Wednesday, April 23!
Registration Is now closed for the 2025 Integration bee.
An Integration Bee is like a spelling bee but competitors do a type of calculus problem by finding definite and indefinite integrals.
Questions? Contact Dr. Stacey Muir (stacey.muir@scranton.edu).
2025 Integration Bee Schedule
The Bee will be held in the DeNaples Centers Ballroom (fourth floor). For directions and information on parking, click here for campus map .
- 4:15 – 4:30 pm
- Sign in
- 4:30 – 4:45 pm
- Review of rules
- 4:45 – 5:25 pm
- First Round: Definite Integration Competition
- 5:25 – 6:00 pm
- Dinner
- 5:45 – 6:00 pm
- Raffle prizes and certificates of participation will be awarded
- 6:00 – 7:30 pm
- Final Round: Indefinite Integration Bee
THANKS TO OUR SPONSORS
Registration
Registration for the 2025 Integration Bee is closed.
Contact Dr. Stacey Muir if you need to cancel your registration.Please be aware if you are under the age of 18 at the time of the competition on April 23, 2025, you must be accompanied by a parent, guardian, or faculty chaperone. Additionally, you will need to provide the name and email of the person accompanying you to complete your registration. A guardian, parent, or faculty member may chaperone more than one student.
School vans and other passenger vehicles can be accommodated. Please contact Mrs. Kover ( noemi.kover@scranton.edu) to arrange.
Rules
No electronic devices (e.g. calculators, phones, smart watches, etc.), printed materials, or notes will be allowed during the competition. No coaching during either of the two rounds is permitted. We will supply scratch paper. The competitors will abide by the following rules.
Round One: Definite Integration Competition
During Round One, participants will be evaluating the same definite integrals. Each participant will be given an answer sheet and will have 2 minutes to complete a definite integral. Each definite integral will be displayed on a screen at the front of the room with a timer. By the end of the given time, participants must write a simplified answer on the answer sheet to be considered for correctness. At the end of the given time, all hands must go up and judges will grade the result immediately. It is at the discretion of the judges to disqualify an answer not written before the given time is over. This will repeat for 15 definite integrals. For each correct answer, participants will receive one raffle ticket to be entered for prizes to be drawn after dinner.
Five participants will advance to the Final Round. Those moving on will be determined by the total number of correct definite integrals. Each definite integral will be weighted though. Only in the event of a tie for the cutoff score for the Final Round will the weights be used to break the ties with those earning a higher weighted score moving on. If after this, there is still a tie for the cutoff score, we will use a second tiebreaker (explained below) for all participants at the cutoff score. Everyone moving into the Final Round will receive $25.
Tiebreaker
A Tiebreaker round will be completed separately at 5:40 p.m. as needed. All participants remaining in the tiebreaker will be required to complete the same indefinite integral in a given time. At the end of the time, if the number of those answering correctly is larger than the minimum number of spots and smaller than the maximum number of spots left available to advance to the Final Round, they will move on and the tiebreaker is over. If the number of those answering correctly exceeds the maximum number to advance, all those answering correctly will continue to the next tiebreaker problem. If the number of those answering correctly is fewer than the minimum to go on, they will advance to the Final Round and all those answering incorrectly will continue to the next tiebreaker problem.
Final Round: Indefinite Integration Bee
This part of the competition will be conducted in rounds. Each contestant remaining at the beginning of a round will attempt an integral in a given amount of time one at a time.
Contestants will be given an indefinite integral one at a time to be attempted on a whiteboard or chalkboard in the front of the room in a given amount of time (approximately 2 – 3 minutes *). If a contestant answers correctly before time expires, the contestant remains in the competition. Once every remaining contestant has attempted one problem, a round is completed. If during any round, all contestants are unable to complete the problem correctly in the time allotted, all contestants will remain in the competition for another round. The last person ** remaining wins an additional $75 and will be crowned the Integration Champion!
Past problems
Previous Integration Bee Problems
- 2024 High School Round One
- 2024 High School Final Round
- 2023 High School Round One
- 2019 High School Round One
- 2019 High School Final Round
- 2018 High School Round One
- 2018 High School Final Round
- 2017 High School Round One
- 2017 High School Final Round
- 2016 High School Round One
- 2016 High School Final Round
- 2015 High School Round One
- 2015 High School Final Round
- 2014 High School Round One
- 2014 High School Final Round
- 2013 High School Round One
- 2013 High School Final Round
- 2012 High School Round One
- 2012 High School Final Round
- 2017 College Round One
- 2017 College Final Round
- 2016 College Round One
- 2016 College Final Round
- 2015 College Round One
- 2015 College Final Round
- 2014 College Round One
- 2014 College Final Round
- 2013 College Round One
- 2013 College Final Round
- 2012 College Round One
- 2012 College Final Round
The countdown timer used in these files functions properly only on select pdf readers, e.g., PDF-XChange Editor and Adobe Acrobat Reader DC work.
Types of Integrals
Integrands will only be real-valued functions of a single variable. The organizers will have full discretion as to what integrals will be issued throughout the contest and to the correctness of the answer.
The integration techniques or integrands that may be employed for the high school division are
- basic functions such as power, trigonometric, exponential, and log functions
- algebraic manipulations to rewrite the integrand
- u-substitution
- integration by parts
During the Final Round, arbitrary constants will not be considered in the evaluation.