Organic Chemistry Module
Greening Across the Chemistry Curriculum English | Versión en Español  | Versão em Português (Brasil)
| Versão em Português (Brasil) 
A Green Chemistry Module
Suggested Use: An organic chemistry course (both lecture and lab) during a discussion of various reactions (substitution, elimination, addition, rearrangements, etc.)
ATOM ECONOMY: A Measure of the Efficiency of a Reaction
Michael C. Cann, Chemistry Department, University of Scranton
michael.cann@scranton.edu
Efficiency of a Reaction
Percentage Yield
Although the efficiency of a reaction can be measured in many ways, by far the most common way is to calculate the yield (percentage yield). Students are often required, especially in laboratory, to determine the theoretical yield based upon the limiting reagent and then to calculate the percentage yield based upon the ratio of the actual yield/theoretical yield X 100. In general organic chemists consider yields of 90% or better as excellent while 20% or less are poor.
Theoretical yield = (moles of limiting reagent)(stoichiometric ratio; desired product/limiting reagent)(MW of desired product)
Percentage yield= (actual yield/theoretical yield) X 100
In order to illustrate the calculation of the percentage yield (and the measurement of the efficiency of a reaction) consider the following acid promoted nucleophilic substitution reaction. A typical procedure1 for this reaction begins with dissolving
Equation 1a
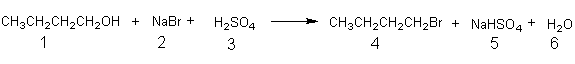
1.33 g of sodium bromide (2) in 1.5 mL of water, followed by addition of 0.80 mL of 1-butanol (1) and 1.1 mL (2.0 g) of concentrated sulfuric acid (3). The following Reagents Table (Table 1) and Desired Product Table (Table 2) can then be
Table 1 Reagents Table
| Reagent | MW | Weight Used (g) |
Moles Used | Theoretical Moles Needed |
Density | Bp (oC) |
| 1 C4H9OH | 74.12 | 0.80 | 0.0108 | 0.0108 | 0.810 | 118 |
| 2 NaBr | 102.91 | 1.33 | 0.0129 | 0.0108 | ||
| 3 H2SO4 | 98.08 | 2.0 | 0.0200 | 0.0108 | 1.84 |
Table 2 Desired Product Table
| Compound | MW | Theoretical Yield (Moles) | Theoretical Yield (Grams) |
Actual Yield (Grams) | % Yield | Density | Bp (oC) |
| 4 C4H9Br | 137.03 | 0.011 | 1.48 (100%) | 1.20 | 81 | 1.275 | 101.6 |
setup. Dividing the weight of each reactant that is used, by the molecular weight of the reactant, gives the number of moles of each reagent used. From the stoichiometry of the reaction (Equation 1a) it is clear that one mole of each reactant is required to produce one mole of product (1-bromobutane) and since 1-butanol (0.0108 mole) is used in the smallest amount it is the limiting reagent. Calculation of the (as shown below) theoretical yield of 1-bromobutane gives 1.48 g. This means that using the
Theoretical yield = (moles of limiting reagent)(stoichiometric ratio; desired product /limiting reagent)(MW of desired product)
= (moles of 1-butanol)(stoichiometric ratio; 1-bromobutane/1-butanol)(MW of 1-bromobutane)
=(0.0180 mole)(1 mole / 1 mole)(137.03 g/mole)
=1.48 g
above quantities of reagents the maximum amount (assuming 100% yield) of 1-bromobutane that can be produced is 1.48 g. In fact no reaction ever proceeds with 100% yield due to such factors as the formation of side products, incomplete conversion of the starting materials, loss upon workup of the reaction mixture, and loss upon isolation and purification of the desired product. This reaction typically produces actual yields of 1-1.2 g. Assuming that the actual yield is 1.20 g calculation of the % yield is as follows. Thus 81% of the theoretical yield is actually isolated, which is a very respectable yield, that would please most chemists.
Percentage yield= (actual yield/theoretical yield) X 100
= (1.20 g/1.48 g) X 100 = 81%
Atom Economy in a Substitution Reaction
As indicated previously most chemists have traditionally measured the efficiency of a reaction by the percentage yield, however this only tells part of the story. If one considers the above reaction where a total of 4.13 g of reactants (0.8 g of 1-butanol, 1.33 g of NaBr and 2.0 g of H2SO4) was used, and that at best this reaction will only yield 1.48 g of the desired product, the question might be asked "what happens to the bulk (4.13 g -1.48 g = 2.7 g) of the mass of reactants?". The answer is they end up in side products (NaHSO4 and H2O) that may be unwanted, unused, toxic and/or not recycled/reused. The side products are oftentimes treated as wastes and must be disposed of or otherwise treated. At best only 36% (1.48 g/4.13 g X 100) of the mass of the reactants end up in the desired product. If the actual yield is 81% then only 29% (.81 X .36 X 100) of the mass of the reactants actually ends up in the desired product!
In an effort to foster awareness of the atoms of reactants that are incorporated into the desired product and those that are wasted (incorporated into undesired products), Barry Trost developed the concept of atom economy.2 In 1998 Trost was awarded aPresidential Green Chemistry Challenge Award for the concept of atom economy. In light of the concept of atom economy, the above acid promoted nucleophilic substitution must now be reconsidered. In Equation 1b we have illustrated the atom economy of this reaction by showing all of the reactant atoms that are incorporated into the desired product in green,
Equation 1b
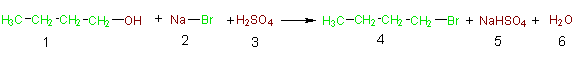
while those that are wasted are shown in brown. Likewise the atoms of the desired product are in green and the atoms composing the unwanted products are in brown. Table 3 provides another view of the atom economy of this reaction. In columns 1 and 2 of this table, the formulas and formula weights (FW) of the reactants are listed. Shown in green (columns 3 and 4) are the atoms and weights of the atoms of the reactants that are incorporated into the desired product (4), and shown in brown (columns 5 and 6) are the atoms and weights of atoms of the reactants that end up in unwanted side products. Focusing on the last row of this table it can be seen that of all the atoms of the reactants (4C, 12H, 5O, 1Br, 1Na and 1S) only 4C, 9H, and 1Br are utilized in the desired product and the bulk (3H, 5O, 1Na, 1S) are wasted as components of unwanted products. This is an example of poor atom economy! A logical extension of Trost's concept of atom economy is to calculate
Table 3 Atom Economy of Equation 1
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 1 C4H9OH | 74 | 4C,9H | 57 | HO | 17 |
| 2 NaBr | 103 | Br | 80 | Na | 23 |
| 3 H2SO4 | 98 | _____ | 0 | 2H,4O,S | 98 |
| Total 4C,12H,5O,BrNaS |
275 | 4C,9H,Br | 137 | 3H,5O,Na,S | 138 |
the percentage atom economy.3 This can be done by taking the ratio of the mass of the utilized atoms (137) to the total mass of the atoms of all the reactants (275) and multiplying by 100. As shown below this reaction has only 50% atom economy.
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (137/275) X 100 = 50%
Thus at best (if the reaction produced 100% yield) then only half of the mass of the reactants would be incorporated into the desired product while the rest would be wasted in unwanted side products.
OPTIONAL MATERIAL
The following optional material deals with two new terms called the "experimental" atom economy, and the "Percentage Yield X Experimental Atom Economy". These two terms further elucidate the efficiency of a reaction however this material may be omitted. To skip the optional material and return to the discussion of the atom economy and other environmental considerations click here.
In reality if the reaction is performed according to the quantities indicated in Table 1 the atom economy would be even less. This is a result of the fact that in Table 3 it was assumed the stoichiometric amounts of each reactant (1:1:1), as indicated in Equation 1b, would be consumed in this reaction. In fact the amounts of reactants 1, 2 and 3 that were used in the actual reaction, as shown in Table 1, are 0.0108:0.0129:0.0200 = 1:1.11:1.85. Table 4 illustrates a term that we have coined the "experimental" atom economy, which is based on the actual quantities of reagents used in the experiment. Table 4 is similar to Table 3 with the exception that
Table 4 Experimental Atom Economy of Equation 1: Based on Actual
Quantities of Reagents Used
| Reagents Formula | Weight of Reagent (FW X moles used) |
Utilized Atoms | Weight of Utilized Atoms (FW X moles) | Unutilized Atoms |
Weight of Unutilized Atoms (FW X moles) |
| 1 C4H9OH | 74.0 X .0108 = .80 | 4C,9H | 57 X .0108= .62 | HO | 17 X .0108=.18 |
| 2 NaBr | 103 X .0129=1.33 | Br | 79.9X .0129=1.03 79.9X .0108=0.86 excess 0.17 |
Na | 23 X .0129=.30 excess 0.17 subtotal 0.47 |
| 3 H2SO4 | 98 X .0200= 2.0 | _____ | 0.00 | 2H,4O,S | 98.1 X .0200=1.96 |
| Total 4C,12H,5O,BrNaS |
4.13 | 4C,9H,Br | 1.48 | 3H,5O,Na,S | 2.61 |
the excess of any reagents that are used is accounted for. In this case the NaBr (reagent 2) is used in excess (.0129 mole) as compared to the 1-butanol (.0108 mole), which is the limiting reagent. Thus in Table 4 in the fourth column where the mass of the reagents that are actually used is calculated, it can be seen that even though the bromine (from the NaBr) is utilized in the desired product there is an excess of this element and therefore the excess must be added into the unutilized weight of reagents. A term we have coined called the Percentage Experimental Atom Economy can now calculated. The percentage experimental atom economy is simply the expected mass of the reagents that are utilized in the desired product (which works out to be the same as the theoretical yield), divided by the actual total mass of all the reagents used in the experiment. In Table 4 we see in column 4, row 4, the utilized mass is 1.48 g and the total mass of the reagents is 4.13 g (row 4, column 2) thus the % experimental atom economy is (1.48/4.13 X 100) 36%. This is of course nothing more than the same calculation that was
% Experimental Atom Economy = (mass of reactants utilized in the desired product/total mass of all reactants) X 100
= (theoretical yield/total mass of all reactants) X 100
= (1.48 g/4.13 g) X 100 = 36%
performed at the beginning of the discussion of atom economy and this represents the maximum % of the mass of the reactants that can be incorporated into the desired product. Although the same conclusion was obtained before a discussion of the concept of atom economy, it is helpful to build a table such as Table 4 so that one can see which reagent(s) are leading to the poor atom economy.
Rather than consider the percentage yield and (experimental) atom economy separately, an even better indication of the efficiency of a reaction is to consider a combination of the two. We have coined the term Percentage Yield X Experimental Atom Economy (%PE .EAE) to illustrate this. This is calculated as follows:
% Yield X Experimental Atom Economy = (actual yield/theoretical yield) X (mass of reactants utilized in the desired
product/total mass of all reactants) X 100
%PE .EAE= (actual yield/theoretical yield) X (theoretical yield/total mass of all reactants)
X 100
= (actual yield/total mass of all the reactants) X 100
= (1.20 g/4.13 g) X 100
= 29%
Notice, that in this calculation, the theoretical yield cancels leaving the ratio of the actual yield to the total mass of all the reactants X 100. If again one assumes that the actual yield is 1.20 g and this is divided by 4.13 g (total mass of all reactants from Table 4) then the % Yield X Experimental Atom Economy is only 29% (previously we arrived at this same conclusion in a much less formal way; see the first paragraph of this section). This means that only 29% of the total mass of all the reactants is actually isolated in the desired product while 71% is wasted! Measuring the efficiency of a reaction in this manner puts it into an entirely new perspective. While many chemists would consider a yield of 81% to be a very acceptable, not many would consider the isolation of only 29% of the mass of the reactant atoms in the desired product to be satisfactory.
This concludes the optional material
Other Environmental Considerations
Although consideration of both the atom economy and the % yield, gives a much better measure of the efficiency of a reaction and its environmental acceptability, other factors need to be considered. To prompt a discussion of the environmental acceptability of a reaction it is prudent to study some of the Twelve Principles of Green Chemistry (see below). Principle numbers 1 and 2 are directly addressed by atom economy and yield.
THE TWELVE PRINCIPLES OF GREEN CHEMISTRY4
1. It is better to prevent waste than to treat or clean up waste after it is
formed.
2. Synthetic methods should be designed to maximize the incorporation
of all materials used in the process into the final product.
3. Wherever practicable, synthetic methodologies should be designed to
use and generate substances that possess little or no toxicity to human
health and the environment.
4. Chemical products should be designed to preserve efficacy of function
while reducing toxicity.
5. The use of auxiliary substances (e.g. solvents, separation agents, etc.)
should be made unnecessary whenever possible and, innocuous when
used.
6. Energy requirements should be recognized for their environmental and
economic impacts and should be minimized. Synthetic methods
should be conducted at ambient temperature and pressure.
7. A raw material feedstock should be renewable rather than depleting
whenever technically and economically practical.
8. Unnecessary derivatization (blocking group, protection/deprotection,
temporary modification of physical/chemical processes) should be
avoided whenever possible.
9. Catalytic reagents (as selective as possible) are superior to
stoichiometric reagents.
10. Chemical products should be designed so that at the end of their
function they do not persist in the environment and break down into
innocuous degradation products.
11. Analytical methodologies need to be further developed to allow for
real-time in-process monitoring and control prior to the formation of
hazardous substances.
12. Substances and the form of a substance used in a chemical process
should chosen so as to minimize the potential for chemical accidents,
including releases, explosions, and fires.
Principles 3 and 4 deal with the toxicity of all substances used in a reaction including the reactants and products. Considering Equation 1 it is clear that the two hydrogens and one oxygen that go into the formation of water are wasted. However if a reaction is going to form a waste product, then water is about as environmentally benign (nontoxic and presents no disposal problem if it is pure) as can be imagined. However all the products and the reactants should be evaluated for their toxicity. Excellent examples of the formation of less toxic products that have the same efficacy, are seen in new pesticides developed by Rohm and Haas for controlling insects and for controlling marine fouling organisms. Each of these new examples of pesticides have won a Presidential Green Chemistry Challenge award.
Principle #5 prompts the consideration of auxiliary substances (solvents, separation agents, drying agents etc.) that are used in reactions and syntheses. Although water is used as the solvent (an environmental plus5), in a typical experimental procedure1 to carry out Equation 1, workup of the product (1-bromobutane) after distillation requires 1 mL of concentrated sulfuric acid, 1 mL of 3M sodium hydroxide, anhydrous calcium chloride, 1 mL of ethanol, 1 mL of acetone and 2 mL of p-xylene all to isolate only 1-1.2 g of 1-bromobutane! It is thus clear that the waste generated from these auxiliary substances is significant and exceeds the amount of waste (at least from a mass point of view) that is generated directly from the reaction. Many organic reactions utilize large amounts of organic solvents which are frequently toxic. These solvents often find their way into the water, soil and air resulting in significant pollution of the environment. Efforts are underway to replace organic solvents with water, carbon dioxide and room temperature ionic liquids. In fact Joseph DeSimone of the University of North Carolina has been awarded a Presidential Green Chemistry Challenge award for his work in developing surfactants for liquid and supercritical carbon dioxide. As a result of Dr. DeSimone's efforts, a process using liquid carbon dioxide has been developed for the dry cleaning of clothes. This process recycles the carbon dioxide, that is obtained as waste from other chemical procedures and allows for the replacement of perchloroethylene, the health effects of which have come into question.
Principle #6 considers the energy requirements of a reaction. It is preferable to perform reactions at ambient temperature and pressure, however many chemical reactions require heating and/or cooling and pressures other than that of the surroundings. This necessitates the use of some energy source and most often this energy source is a fossil fuel. In order to perform the reaction and isolate the 1-bromobutane formed in Equation 1, a reflux and two distillations are required. Thus the energy requirements of this reaction are significant.
Most organic starting materials, such as the 1-butanol in Equation 1, are ultimately derived from crude oil, a nonrenewable (depleting) resource. Principle #7 urges us to consider whether these starting materials can be derived from renewable resources. Renewable resources generally means biological and plant based materials (biomass). Carbon dioxide and methane are also generally considered to be renewable since they can be generated from both natural and synthetic methods. Work by Draths and Frost at Michigan State, Mark Holtzapple at Texas A&M, and Biofine Corporation to create chemical feedstocks from biomass have all won Presidential Green Chemistry Challenge awards.
Some may mistakenly refer to the reaction described in Equation 1 as an acid catalyzed reaction when in fact it is actually an acid promoted reaction. This is a result of the fact that the sulfuric acid in this reaction is required in stoichiometric, not catalytic amounts. As principle #9 indicates reagents used in catalytic amounts are preferable to reagents used in stoichiometric amounts. Since one mole of sulfuric acid is required for the loss of every water molecule in this reaction, then only stoichiometric quantities of this reagent will suffice. However even if stoichiometric amounts are used then recovery/recycling/reuse of unwanted products should take place whenever this is feasible. Significant strides have recently been made to develop reactions that are promoted by nontoxic and recoverable catalysts. A biocatalytic process discovered and developed by Lilly Research Laboratories for producing a potential anticonvulsant drug has won a Presidential Green Chemistry Challenge award.
Atom Economy in Elimination Reactions
In all of the remaining discussions of the efficiency of a reaction, the discussion will be limited to the atom economy based on the stoichiometry of the reaction. When one encounters these reactions in the laboratory it may also be prudent to calculate the atom economy based on the quantities of the reagents used (experimental atom economy). In addition one may also want to consider matters such as toxicity, energy use, the use of auxiliary substances, catalytic versus stoichiometric reagents and renewable versus nonrenewable feedstocks.
In the substitution reaction above (Equation 1a) it was revealed that the poor atom economy resulted from the fact that the atoms of the leaving group (OH) that is being replaced, the counterion (sodium) of our nucleophile (bromide), and the sulfuric acid that is required for this reaction all are wasted in forming unwanted products in this reaction. By virtue of the fact that elimination reactions require only the loss of atoms (while gaining none) from the reactant, means that elimination reactions are in general even worse, in terms of their atom economy, than substitution reactions.
As an example consider the atoms of the following elimination reaction. Base promoted dehydrohalogenation of alkyl halides is a common method of producing alkenes from alkyl halides via elimination. In Equation 2 the formation of methyl propene is accomplished by the reaction of 2-bromo-2-methylpropane (7) with sodium ethoxide (8). In this reaction, the atoms of the reactants that are incorporated into the desired product (9) and the atoms of the desired product are indicated in green, while the unutilized atoms of the reactants are shown in brown as are the atoms in the unwanted products of the reaction. Table 5 illustrates the atom economy of this reaction and calculation of the % atom economy gives a very poor 27%. The poor atom
Equation 2
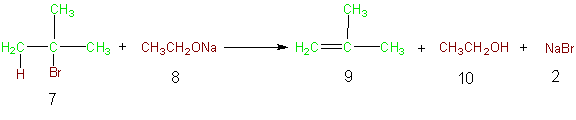
Table 5 Atom Economy Equation 2
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 7 C4H9Br | 137 | 4C,8H | 56 | HBr | 81 |
| 8 C2H5ONa | 68 | ____ | 0 | 2C,5H,O,Na | 68 |
| Total 6C,14H,O,Br,Na |
205 | 4C,8H | 56 | 2C,6H,O,Br,Na | 149 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (56/205) X 100 = 27%
economy is a result not only of the loss of the HBr but also because this is a base promoted reaction and all of the atoms of the sodium ethoxide base are found in unwanted side products.
Atom Economy in Addition Reactions
Because addition reactions in general lead to the incorporation of all the atoms of the reactants into the final desired products, addition reactions result in high atom economy. From an atom economy point of view, addition reactions are thus environmentally preferable to elimination and substitution reactions. As a case in point consider the following addition of hydrogen bromide to methyl propene. In this example all the atoms of the reactants (9 and 11) are shown in green since all of these atoms are utilized in the final desired product (7). The table of atom economy (Table 6) and the calculation of 100% atom economy further emphasize the excellent atom economy of this reaction.
Equation 3
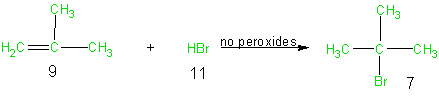
Table 6 Atom Economy Equation 3
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 9 C4H8 | 56 | 4C,8H | 56 | ____ | 0 |
| 11 HBr | 81 | HBr | 81 | ____ | 0 |
| Total 4C,9H,Br |
137 | 4C,9H,Br | 137 | ____ | 0 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (137/137) X 100 = 100%
Atom Economy in Rearrangement Reactions
Rearrangement reactions involve reorganization of the atoms of a molecule. Because neither elimination, addition or substitution of atoms is taking place, in the molecule undergoing rearrangement, the atom economy of rearrangement reactions is 100% and they are environmentally preferable reactions from an atom economy standpoint. To illustrate this, consider the acid catalyzed rearrangement of 3,3-dimethyl-1-butene (12) to 2,3-dimethyl-2-butene (13). In this case the atoms of the reactant 12 are all shown in green since they are all incorporated into the desired product 13. As in the previous examples one can set up an atom economy table and calculate the % atom economy. Although the acid (H+) used in this reaction is not incorporated into the desired product it is used only in catalytic amounts and therefore indicated in black (not green or brown) and it is not considered in the atom economy table or the calculation of the atom economy. As was predicted above for rearrangements, the % atom economy of this reaction is 100%.
Equation 4
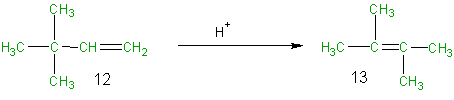
Table 7 Atom Economy Equation 4
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 12 C6H12 | 84 | 6C,12H | 84 | ____ | 0 |
| Total 6C,12H |
84 | 6C,12H | 84 | ____ | 0 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (84/84) X 100 = 100%
Virtually all organic reactions fall into the categories of substitution, addition, elimination or rearrangement reactions. From the perspective of atom economy, addition and rearrangement reactions are environmentally preferable, with substitution reactions next, while eliminations are the least environmentally preferable. As one encounters reactions, in the study of chemistry, one should examine each reaction from the point of not only the yield, but also the atom economy of the reaction.
Atom Economy in Syntheses
The concept of atom economy can also be applied to syntheses. To determine the atom economy of a synthesis, one simply must determine which of the atoms of the reactants for all steps in the synthesis are incorporated into the desired final product.
The Synthesis of Ethylene Oxide
To illustrate the concept of atom economy in a synthesis, consider the industrial synthesis of ethylene oxide (19). Ethylene oxide is a feedstock in the synthesis of ethlyene glycol (used in antifreeze), ethoxylates (surfactants), and glycol ethers and polymers (such as PET). Approximately 8,800 million pounds of this compound are prepared per year on a global basis. The classical synthesis (Scheme 1) of ethylene oxide (19) is a two-step synthesis and is known as the chlorohydrin route. The atoms of the reactant atoms that are incorporated into the final desired product (19), and the atoms of the desired product are
Scheme 1 The Clorohydrin Route to Ethylene Oxide
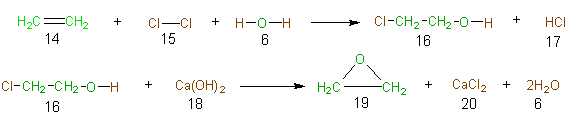 green, and those atoms of the shown in green, while the atoms of the reactants that find their way into unwanted products (along with the atoms of the unwanted products) are shown in brown. Table 8 is the table of atom economy for the chlorohydrin route to ethylene oxide and allows one to calculate the poor atom economy (23%) of this route.
green, and those atoms of the shown in green, while the atoms of the reactants that find their way into unwanted products (along with the atoms of the unwanted products) are shown in brown. Table 8 is the table of atom economy for the chlorohydrin route to ethylene oxide and allows one to calculate the poor atom economy (23%) of this route.
Table 8 Atom Economy of Scheme 1, The Clorohydrin Route to Ethylene Oxide
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 14 C2H4 | 28 | 2C,4H | 28 | _____ | 0 |
| 15 Cl2 | 71 | _____ | 0 | 2Cl | 71 |
| 6 H2O | 18 | O | 16 | 2H | 2 |
| 18 Ca(OH)2 | 72 | _____ | 0 | Ca,4H,2O | 72 |
| Total 2C,8H,3O,Ca,2Cl |
189 | 2C,4H,O | 44 | 6H,2O,Ca,2Cl | 145 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (44/189) X 100 = 23%
A catalytic route (Scheme 2) to ethylene oxide from ethylene has been developed. In this one step synthesis the only other reagent, aside from ethylene, that is used is 1/2 mole of oxygen. As can be seen from Scheme 2 and Table 9 all of the reagent atoms are incorporated into the desired final product and thus this synthesis has 100% atom economy. The atom economy of 100% for this reaction is not suprising since this is an addition reaction and as was indicated preciously, addition reactions have 100% atom economy.
Scheme 2 The Catalytic Route to Ethylene Oxide
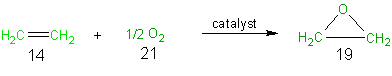
Table 9 Atom Economy of Scheme 2, The Catalytic Route to Ethylene Oxide
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 14 C2H4 | 28 | 2C,4H | 28 | _____ | 0 |
| 21 1/2 O2 | 16 | O | 16 | _____ | 0 |
| Total 2C,4H,1Ol |
44 | 2C,4H,O | 44 | _____ | 0 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (44/44) X 100 = 100%
The Synthesis of Ibuprofen
The Boots Company Synthesis of Ibuprofen
Ibuprofen is the active ingredient in a number of brand name products including Advil, Motrin and Nuprin. Ibuprofen acts as an analgesic (pain reliever) and is also effective as a Non Steroidal Anti-Inflammatory Drug (NSAID). NSAIDs reduce inflammation from such conditions as arthritis, osteoarthritis and rheumatism. Ibuprofen is referred to as a non steroidal anti-inflammatory drug since ibuprofen is not a member of the steroid family of compounds.
The world production of ibuprofen exceeds 30 million pounds per year. The Boots Company PLC of Nottingham, England first patented the synthesis of ibuprofen in the 1960's (U.S. Patent 3,385,886) and this has served as the main method of synthesis for many years. The Boot's synthesis of ibuprofen is a six-step synthesis and is shown in Scheme 3. As usual all the
Scheme 3 The Boots Synthesis of Ibuprofen
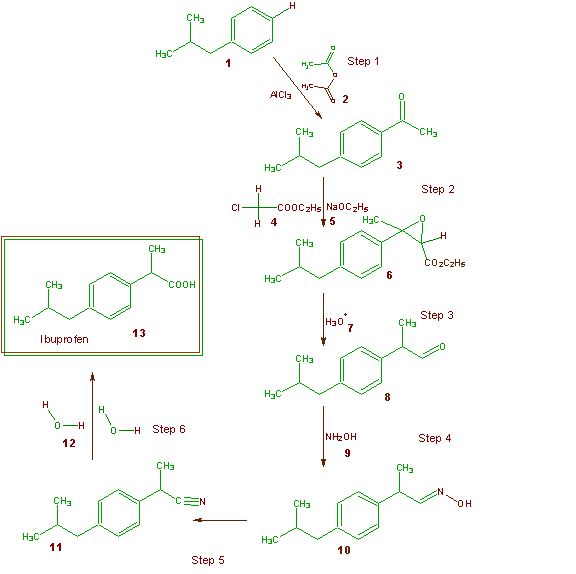
atoms of each reagent that are incorporated into the final desired product (ibuprofen) are shown in green and those that end up in unwanted products are shown in brown. Table 10 illustrates the atom economy of the Boots Company synthesis and allows one to calculate a percentage atom economy of 40%. As was indicated above about 30 million pounds of ibuprofen are manufactured on a yearly basis. If all the world's supply of ibuprofen were manufactured by the Boots process, then this would generate about 35 million pounds of waste!
Table 10 Atom Economy of Scheme 3, the Boots Company Synthesis of Ibuprofen
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 1 C10H14 | 134 | 10C,13H | 133 | H | 1 |
| 2 C4H6O3 | 102 | 2C,3H | 27 | 2C,3H,3O | 75 |
| 4 C4H7ClO2 | 122.5 | C,H | 13 | 3C,6H,Cl,2O | 109.5 |
| 5 C2H5ONa | 68 | _____ | 0 | 2C,5H,O,Na | 68 |
| 7 H3O | 19 | _____ | 0 | 3H,O | 19 |
| 9 NH3O | 33 | _____ | 0 | 3H,N,O | 33 |
| 12 H4O2 | 36 | H,2O | 33 | 3H | 3 |
| Total 20C,42H,N,10O, Cl,Na |
514.5 | Ibuprofen 13C,18H,2O |
Ibuprofen 206 |
Waste Products 7C,24H,N,8O, Cl,Na |
Waste Products 308.5 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (206/514.5) X 100 = 40%
The BHC Company Synthesis of Ibuprofen
In the eighties ibuprofen was approved for over-the-counter use and the Boots Company patent expired. Recognizing the financial opportunities that the manufacture and sales of this drug could offer, several companies embarked upon setting up facilities and developing new methods for the preparation of ibuprofen. The Hoechst Celanese Corporation (Somerville, NJ; now know as the Celanese Corporation) discovered a new three-step synthesis of ibuprofen. Together with the Boots Company they formed the BHC Company to prepare (by the new synthesis) and market ibuprofen. The BHC Company synthesis is show in Scheme 4 with the utilized atoms in green and the untutilized atoms in brown. The atom economy is further illustrated in Table 11 and calculation of the % atom economy gives 77%, a significant improvement over the 40% of the
Scheme 4 The BHC Company Synthesis of Ibuprofen
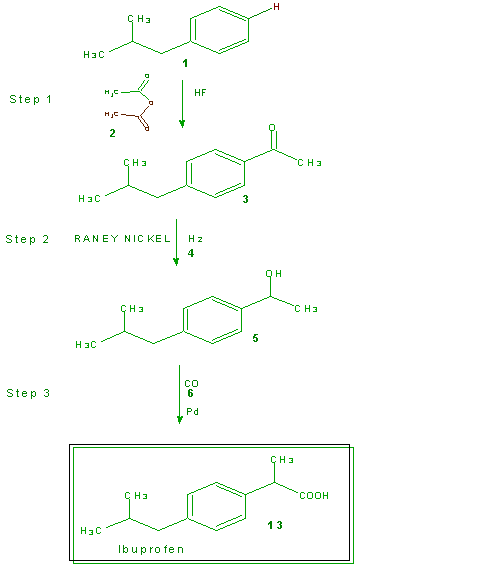
Table 11 Atom Economy of Scheme 4, the BHC Company Synthesis of Ibuprofen
| Reagents Formula | Reagents FW | Utilized Atoms | Weight of Utilized Atoms |
Unutilized Atoms |
Weight of Unutilized Atoms |
| 1 C10H14 | 134 | 10C,13H | 133 | H | 1 |
| 2 C4H6O3 | 102 | 2C,3H,O | 43 | 2C,3H,2O | 59 |
| 4 H2 | 2 | 2H | 2 | _____ | 0 |
| 6 CO | 28 | CO | 28 | _____ | 0 |
| Total 15C,22H,4O |
266 | Ibuprofen 13C,18H,2O |
206 | Waste Products 2C,3H,2O |
60 |
% Atom Economy = (FW of atoms utilized/FW of all reactants) X 100
= (206/266) X 100 = 77%
Boots Company process. The atom economy of the BHC Company process jumps to >99% if one considers that the acetic acid generated in Step 1 is recovered and used.
Not only does the BHC Company process offer a dramatic improvement in the atom economy it offers other environmental advantages. These include a three-step catalytic process vs. the six-step Boots Company process that requires auxiliary reagents in stoichiometric amounts. For example, the first step in each process yields the same product (3) from the same reactants (1 and 2). However, the Boots Company process utilizes aluminum trichloride in stoichiometric amounts (not accounted for in Table 10) while the BHC Company process uses HF in catalytic amounts that is recovered and reused repeatedly. The aluminum trichloride produces large amounts of aluminum trichloride hydrate as a waste product which is generally landfilled. The nickel and palladium catalysts used in Steps 2 and 3 of the BHC Company process are also recovered and reused.
Because the BHC Company process is only three steps (vs. six steps for the Boots Company process) and it has a much improved atom economy, it not only results in a dramatic decrease in the waste produced it also allows for a greater throughput (more ibuprofen in less time and with less equipment). These factors translate into economic benefits for the company as a result of the fact that less money is required to deal with the waste that is generated and less capital expenditure is required to produce the same amount of ibuprofen. Thus not only does the environment benefit, but the company bottom line is strengthened and good public relations can be reaped as a result of a greener process.
For the development of this synthesis of ibuprofen, the BHC company won a prestigious Presidential Green Chemistry Challenge award in 1997 and they also won the prominent Kirpatrick Chemical Engineering Achievement Award6 in 1993. The Kirpatrick award is given on a biennial basis by Chemical Engineering magazine and recognizes outstanding group efforts in developing and commercializing process technology.
Summary
Atom economy and experimental atom economy go beyond the calculation of the yield of a reaction and offer a second way to measure the efficiency of a reaction taking into account the utilized and unutilized atoms of the reactants. The percentage atom economy and the percentage experimental atom economy, give one the means to quantify the atom economy, and allows for a quantitative comparison of the atom economy of one reaction (or synthesis) vs. another. Perhaps the best measure of the efficiency of a reaction is to take into consideration both the atom economy and the yield by calculating the percentage yield X experimental atom economy (PE .EAE). Of course in assessing the environmental suitability of a reaction (or synthesis) not only should one consider the efficiency of the reaction but also (as discussed above) other factors such as toxicity, the use of auxiliary substances, energy requirements, feedstock origins, and catalytic vs. stoichiometric reagents.
Questions
1. Define the following terms
a) atom economy
b) % atom economy
c) % yield
d) % experimental atom economy
c) %PE .EAE
2. Which of the terms in question 1 are only meaningful with experimental results? What experimental result is necessary
to make these terms meaningful?
3. Consider the following reactions.
a) Label each reaction as a substitution, elimination, addition or rearrangement.
b) Rewrite each reaction making sure that the reaction is balanced. Show all the reactant atoms that are incorporated into
the desired product, and the atoms of the desired product in green, and all other atoms in brown.
c) Set up a table of atom economy, analogous to Table 3, for each reaction .
d) Calculate the % atom economy of each reaction.
![]()
![]()
![]()
![]()
4. As indicated in reference 3, Roger Sheldon has developed a term very similar to the % atom economy called % atom
utilization. The % atom utilization can be calculated according to the following equation.
% Atom Utilization = (MW of desired product/MW of all products) X 100
a) Compare and contrast this with the % atom economy.
b) With the information given in Schemes 3 and 4 can you calculate the % atom utilization for these syntheses? Explain your
answer.
c) What concept that you learned in freshman chemistry makes the actual percentages calculated for the %
atom utilization, and % atom economy equal (under most circumstances). Prove this by calculating the % atom utilization for
each of the reactions in question 3 and comparing your results to the % atom economy that you calculated in question 3.
5. Consider the following two-step synthesis of 2-pentyne.

a) Rewrite the synthesis making sure that each reaction is balanced. Show all the reactant atoms that are incorporated
into the desired product, and the atoms of the desired product in green, and all other atoms in brown.
c) Set up a table of atom economy for this synthesis analogous to Table 7.
d) Calculate the % atom economy of this synthesis.
e) Calculate the % atom utilization of this synthesis.
.
6. Your instructor will direct you to an experiment in your current laboratory textbook. With regard to this lab:
a) Write a balanced equation showing the atoms of the reactants that are incorporated into the desired product and the
atoms of the desired product in green, and all other atoms in brown.
b) Construct a table of atom economy analogous to Table 3.
c) Calculate the % atom economy.
d) Construct a table of experimental atom economy analogous to Table 4.
e) Calculate the experimental atom economy.
f) Assume that this reaction gives 80% yield. Calculate the %PE .EAE.
g) What are the energy requirements of this reaction?
h) Are there auxiliary substances used in this reaction?
i) What reagents are used in stoichiometric amounts?
j) What reagents are used in catalytic amounts?
7. Supply a mechanism for Step 1 of the BHC Company synthesis of ibuprofen. Is this step acid catalyzed or acid promoted?
8. A third synthesis of ibuprofen, called the ethyl synthesis, is shown below.
a) Rewrite the synthesis showing all the reactant atoms that are incorporated into the desired product, and the atoms of
the desired product in green, and all other atoms in brown.
c) Set up a table of atom economy for this synthesis analogous to Table 7.
d) Calculate the % atom economy of this synthesis.
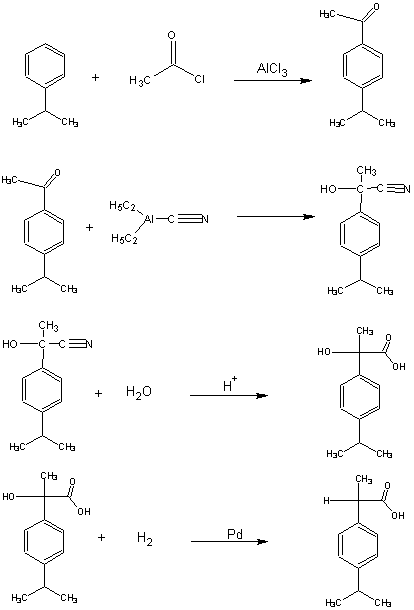
.
References and Notes
1. Williamson, Kenneth L., Macroscale and Microscale Organic Experiments, 2nd ed., D. C. Heath and Co., 1994, 247-252.
2. Trost, Barry M., The Atom Economy-A Search for Synthetic Efficiency. Science 1991, 254, 1471-1477.
3. Cann, Michael C.; Connelly, Marc E. Real-World Cases in Green Chemistry; ACS, Washington, 2000. Roger Sheldon of Delft University has developed a very similar concept called % atom utilization. Sheldon, Roger A. Organic Synthesis-Past, Present and Future. Chem. Ind. (London), 1992, (Dec), 903-906.
4. Anastas, Paul T., and Warner, John C. Green Chemistry Theory and Practice, Oxford University Press, New York, 1998.
5. There is a significant effort underway to reduce the use of organic compounds as solvents for reactions and replacement of these with water or even better yet no solvent at all.
6. Westheimer, T. The Award Goes to BHC. Chem. Eng. 1993, (Dec.), 84-95.